Angular velocity refers to the rate at which an object’s angular position changes over time. It combines two concepts: “angular,” which relates to rotation, and “velocity,” which represents the change in position per unit of time. In essence, angular velocity measures the change in angular position per unit of time.
Imagine a particle, labeled P, moving in a circular path around a center point, labeled Q. As P revolves around Q during a specific time interval (t), it creates an angle at the center (theta). This angle, theta, represents the angular displacement.
Angular velocity, denoted by the symbol omega, is the ratio of angular displacement to time. In other words, omega equals the angle subtended per unit time. If the particle’s direction of motion is counterclockwise, both angular velocity and angular displacement are considered vector quantities, meaning they possess both magnitude and direction. Consequently, the direction of angular displacement aligns with that of angular velocity.
Angular Velocity Formula
The angular velocity formula, represented by the Greek letter omega (ω), is calculated as ω = 2πN / 60 rad/s, where N represents the number of revolutions per minute (rpm). This formula is derived from the linear velocity concept, which is defined as the distance traveled divided by time.
In linear motion, if an object covers two units of distance in one second, its velocity is two units per second. Similarly, we can apply this concept to angular motion, considering the angular displacement per unit of time. In this case, N represents the rpm of a rotating body, meaning the number of complete rotations it makes in a minute. To convert this value to revolutions per second, we need to divide the rpm by 60.
By doing this, we can find the angular velocity of a rotating object, which helps us understand its motion and behavior in various applications, such as engineering, physics, and astronomy. This calculation is essential for designing and analyzing systems involving rotating components, like motors, gears, and wheels.
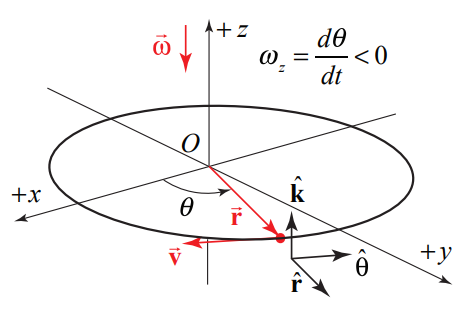
The angular velocity formula can be derived by understanding the relationship between time, revolutions, and degrees. A minute consists of 60 seconds, and revolutions per minute can be converted to revolutions per second by dividing by 60. Therefore, for one complete revolution, the body rotates 360 degrees or 2 pi radians. If N revolutions occur in one minute, then the angular distance covered will be 2 pi N radians. To calculate the angular velocity, we need to consider the time taken for one revolution. Thus, dividing the angular distance covered in one second by the time taken gives us the angular velocity. Therefore, the angular velocity formula can be expressed as omega equals 2 pi N divided by 60 radians per second.
ω= 2πN/60 rad/s
Velocity In Linear Motion
Velocity in linear motion is a measure of the rate of change of displacement with respect to time. It is a vector quantity, which means it has both magnitude and direction. The magnitude of the velocity vector is the speed, which is the distance traveled per unit of time, while the direction of the velocity vector is the direction in which an object is moving. The SI unit of velocity is meters per second (m/s).
There are two types of velocity in linear motion: average velocity and instantaneous velocity. Average velocity is calculated by dividing the displacement by the time interval, while instantaneous velocity is the velocity of an object at a particular instant in time.
In addition, velocity can be positive or negative depending on the direction of motion. If an object is moving in the positive direction, its velocity will be positive, while if it is moving in the negative direction, its velocity will be negative. Finally, the velocity of an object can change due to a change in its speed or direction of motion.
In Angular Motion
If N=rpm=revolutions per minute
N/60=rps=revolutions per second
1 revolution=360 degree=2π radians
Next, 1 minute= (N) revolutions =2π into N radians
Next, 1 second=2πN/60 rad/s
ω= 2πN/60 rad/s
Angular Velocity Unit
The unit of angular velocity is radians per second (rad/s). Radians measure the angle subtended by an arc of a circle equal in length to the radius of the circle, while seconds measure time. Therefore, the unit of radians per second describes the angular distance covered by an object in one second, which is a measure of its angular velocity. In some cases, degrees per second (°/s) may also be used as a unit of angular velocity, but radians per second is the more commonly used unit.
Angular Velocity Symbol
The symbol for angular velocity is the Greek letter omega (ω).
Angular Velocity To Linear Velocity
When an object moves along a circular path of radius r, it undergoes both linear and angular displacement. Linear displacement is the change in position from one point to another on the circular path, while angular displacement is the angle traversed by the object about the center of the circle. The relationship between linear velocity (v) and angular velocity (ω) can be derived by considering the rate of change of linear displacement with respect to time.
If we denote the angular displacement by θ, we can write θ = l/r, where l is the linear displacement. Differentiating both sides with respect to time yields dl/dt = r(dθ/dt), where r is constant. The rate of change of linear displacement (dl/dt) is the linear velocity (v), and the rate of change of angular displacement (dθ/dt) is the angular velocity (ω). Thus, we can write v = ωr.
This relationship between linear and angular velocity is a scalar form. In vector form, we can express the relationship as v = ω x r, where x denotes the cross product of ω and r. Here, r is the radius vector or position vector of the object. Therefore, we can use the equation v = ω x r to relate the linear and angular velocity of an object moving on a circular path of radius r.
Angular Velocity Dimensional Formula
The dimensional formula of angular velocity can be derived using the formula:
ω = θ/t,
where ω is the angular velocity, θ is the angular displacement, and t is the time taken. The dimensional formula of θ is [L][L]⁻¹ = 1, and the dimensional formula of t is [T].
Substituting these values, we get:
[ω] = [θ]/[t] = 1/[T],
which means that the dimensional formula of angular velocity is [T]⁻¹.
In SI units, the unit of angular velocity is radians per second (rad/s), which has the dimensional formula of [M][L][T]⁻². Therefore, we can express the dimensional formula of angular velocity as:
[T]⁻¹ = [M][L][T]⁻².
Note that the dimensional formula of angular velocity does not depend on any other physical quantity apart from time.
Angular Velocity Of The Earth’s Equation
The angular velocity of the Earth can be defined as the rate at which the Earth rotates about its own axis. It is denoted by the symbol ω and has a constant value of approximately 7.2921159 × 10⁻⁵ radians per second (rad/s). The equation for the angular velocity of the Earth can be expressed as:
ω = 2π/T,
where T is the time taken for the Earth to complete one full rotation about its axis. The value of T is approximately 23 hours, 56 minutes, and 4.1 seconds, which is also known as a sidereal day. Substituting this value in the above equation, we get:
ω = 2π/(23 h 56 min 4.1 s) = 7.2921159 × 10⁻⁵ rad/s.
This means that the Earth completes one full rotation about its axis in approximately 23 hours, 56 minutes, and 4.1 seconds, and its angular velocity is approximately 7.2921159 × 10⁻⁵ rad/s. The angular velocity of the Earth is an important parameter in many fields, including astronomy, geophysics, and navigation.
Read Also:Circle of Illumination Diagram, Definition and Concepts
Angular Velocity And Speed
When an object moves along a circular path, its motion can be described in terms of angular velocity. Angular velocity is a measure of how fast an object is rotating around a central point, and it is denoted by the symbol ω. In other words, angular velocity is the rate at which the angle between the object and the central point is changing.
To calculate the angular velocity, we need to know the time taken for each revolution and the angle covered in one revolution. For instance, if an object is moving in a circular path with a frequency of five revolutions per second, it means that it covers 2π radians per revolution. Therefore, the angular velocity can be calculated by multiplying the frequency by the angle covered per revolution. In this case, the angular velocity would be 10π radians per second.
Angular velocity is a vector quantity, meaning that it has both magnitude and direction. However, the direction of the vector depends on the direction of rotation. In a counterclockwise rotation, the angular velocity vector appears to pop out of the page, while in a clockwise rotation, it appears to sink into the page. It is important to note that angular velocity is a pseudo-vector, which means that it behaves like a vector but reverses direction when the coordinate system is flipped.
In summary, angular velocity is an important concept in circular motion that describes the rate of rotation of an object around a central point. It is a vector quantity that is measured in radians per second and is dependent on the direction of rotation.
Angular velocity equation
The equation for angular velocity (ω) can be expressed as the rate of change of angular displacement (θ) with respect to time (t), or:
ω = dθ/dt,
where dθ is the change in angular displacement over a small interval of time dt. Angular displacement is measured in radians, and time is measured in seconds. Therefore, the unit of angular velocity is radians per second (rad/s).
Alternatively, we can derive the equation for angular velocity from the relationship between linear velocity (v) and angular velocity (ω) using the formula:
v = rω,
where r is the radius of the circular path. Rearranging this equation, we can express the angular velocity as:
ω = v/r,
which means that the angular velocity is equal to the linear velocity divided by the radius of the circular path.
These equations can be used to calculate the angular velocity of an object moving in a circular path, given the time taken for each revolution, the angle covered in one revolution, or the linear velocity and radius of the circular path.
Some frequently asked questions
What is the angular velocity in simple terms?
Angular velocity refers to the change in angular displacement of a particle over time. The term “angular velocity” comprises two words: “angular” and “velocity”. The word “velocity” typically denotes the change in displacement with respect to time. In the case of “angular velocity”, the term “angular” refers to the angular displacement of an object, while the term “velocity” refers to the rate of change of that angular displacement over time.
What is called angular speed?
Angular velocity is often used interchangeably with angular speed, even though they have slightly different meanings. Angular velocity is a vector quantity that describes the rate of change of angular displacement with respect to time. While it may seem counterintuitive, the vector associated with angular velocity appears to be popping out of the page. However, this vector is actually a pseudovector, meaning that its direction is dependent on the orientation of the coordinate system.
Is Omega angular velocity?
The symbol for angular velocity is omega (ω).
What is the symbol of angular acceleration?
Angular acceleration is represented by the symbol alpha (α).
What is omega equal to?
The equation that relates angular velocity (ω) to frequency (f) is expressed as follows: ω = 2πf, which means that omega is equal to 2 times pi multiplied by f.
What is the formula of angular velocity?
Angular velocity formula is omega equals to 2 pi N over 60 rad per second. However, we know the velocity formula in linear motion is the general formula; velocity is nothing but the distance by time.
What is omega in physics class 11?
Average angular speed = ΔΘ/Δt. However, angular speed, ω = dΘ / dt. V = w r, in which v – linear speed of particle transferring in a circle of radius r.
